KILOGRAMS and POUNDS - WEIGHTS

Gosh that's heavy. But what does that mean? A very strong adult may not think something is heavy, while a child might think it impossible to move. As we grow older, we come to terms with the weight of everyday objects, such as lifting a kettle, or a bucket of water - our muscles adapt to our environment and the gravitational force that keeps us on our planets surface.  Later, we might pick up a push bike and later still need to push a reluctant motor car. 

This is all very well, but our neghbours in China or France may want to compare weight, to be able to exchange goods (sell or barter). An engineer in the Ukraine will need an accurate way of working with drawings and buying materials to build a boat for a customer in England. We all need to have an agreed way of measuring things to be able to get on in a global community. NK

Nelson says "When calculating mass - this page could help"

The kilogram or kilogramme (symbol: kg) is the SI base unit of mass. The kilogram is defined as being equal to the mass of the International Prototype Kilogram (IPK), which is almost exactly equal to the mass of one liter of water. It is the only SI base unit with an SI prefix as part of its name. It is also the only SI unit that is still defined in relation to an artifact rather than to a fundamental physical property that can be reproduced in different laboratories.

While the weight of objects are often given in kilograms, the kilogram is, in the strict scientific sense, a unit of mass. The equivalent unit of weight is the kilogram-force. Similarly, the avoirdupois pound used in both the Imperial system and U.S. customary units, is a unit of mass and its related unit of weight is the pound-force. The avoirdupois pound is defined as exactly 0.453 592 37 kg, making one kilogram approximately equal to 2.205 avoirdupois pounds.

Many units in the SI system are defined relative to the kilogram so its stability is important. After the IPK had been found to vary in mass over time, the International Committee for Weights and Measures (known by the initials CIPM) recommended in 2005 that the kilogram be redefined in terms of fundamental constants of nature.

The nature of mass

The kilogram is a unit of mass, the measurement of which corresponds to the general, everyday notion of how “heavy” something is. However, mass is actually an inertial property; that is, the tendency of an object to remain at constant velocity unless acted upon by an outside force. An object with a mass of one kilogram will accelerate at one meter/second² (about one-tenth the acceleration of Earth’s gravity) when acted upon (pushed by) a force of one newton (symbol: N).

While the weight of matter is entirely dependent upon the strength of gravity, the mass of matter is constant (assuming it is not traveling at a relativistic speed with respect to an observer). Accordingly, for astronauts in microgravity, no effort is required to hold an object off the cabin floor since such objects naturally hover. However, since objects in microgravity still retain their mass, an astronaut must exert one hundred times more effort to accelerate a 100-kilogram object at the same rate as for a 1-kilogram object.

SI multiples

Because SI prefixes may not be concatenated (united serially) within the name or symbol for a unit of measure, SI prefixes are used with the gram, not the kilogram, which already has a prefix as part of its name. For instance, one-millionth of a kilogram is 1 mg (one milligram), not 1 µkg (one microkilogram). The most common prefixed forms of gram are shown in bold text in the table below.

• When the Greek lowercase “µ” (mu) in the symbol of microgram is typographically unavailable, it is occasionally—although not properly—replaced by Latin lowercase “u”.

• The microgram is often abbreviated “mcg”, particularly in pharmaceutical and nutritional supplement labeling, to avoid confusion since the “µ” prefix is not well recognized outside of technical disciplines. Note however, that the abbreviation “mcg”, is also the symbol for an obsolete CGS unit of measure known as the “millicentigram,” which is equal to 10 µg.

• The unit name “megagram” is rarely used, and even then, typically only in technical fields in contexts where especially rigorous consistency with the units of measure is desired. For most purposes, the term “tonne,” or “metric ton” is instead used.

History

Early definitions

On 7 April 1795, the gram was decreed in France to be equal to “the absolute weight of a volume of pure water equal to a cube of one hundredth of a meter, and to the temperature of the melting ice.” The regulation of trade and commerce required a practical reference standard in addition to the definition based on fundamental physical properties. Accordingly, a provisional kilogram standard was made as single-piece, metallic reference standard one thousand times more massive than the gram.

In addition to this provisional kilogram standard, work was commissioned to determine precisely how massive a cubic decimeter (now defined as one liter) of water is. Although the decreed definition of the kilogram specified water at 0 °C — a highly stable temperature point — the scientists chose to redefine the standard and perform their measurements at the most stable density point: the temperature at which water reaches maximum density, which was measured at the time as 4 °C. They concluded that one cubic decimeter of water at its maximum density was equal to 99.92072% of the mass of the provisional kilogram made earlier that year. Four years later in 1799, an all-platinum standard, the “Kilogramme des Archives,” was fabricated with the objective that it would equal, as close as was scientifically feasible for the day, to the mass of cubic decimeter of water at 4 °C. The kilogram was defined to be equal to the mass of the Kilogramme des Archives and this standard stood for the next ninety years.

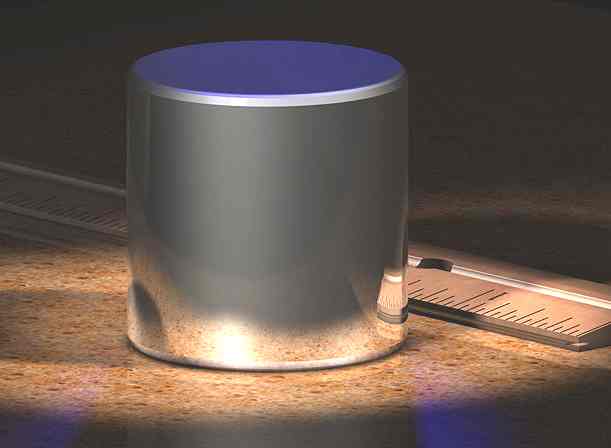
International Prototype Kilogram

Since 1889, the SI system defines the magnitude of the kilogram to be equal to the mass of the International Prototype Kilogram — often referred to in the professional metrology world as the “IPK”. The IPK comprises an alloy of 90% platinum and 10% iridium (by weight) and is machined into a right-circular cylinder (height = diameter) of 39.17 mm to minimize its surface area. The IPK and six of its official copies (its “sister prototypes”) are stored in an environmentally-monitored vault in the basement of the BIPM’s House of Breteuil in Sèvres on the outskirts of Paris. Three independently controlled keys are required to open the vault. Official copies of the IPK were made available to other nations to serve as their national standards. These are compared to the IPK roughly every 40 years.

The IPK is one of three cylinders made in 1879. In 1883, it was found to be indistinguishable from the mass of the Kilogramme des Archives made eighty-four years prior, and formally ratified as the kilogram by the 1st CGPM in 1889. Modern measurements of the density of purified water that has a carefully controlled isotopic composition (known as Vienna Standard Mean Ocean Water) show that a cubic decimeter (one liter) of water at its point of maximum density, 3.984 °C, has a mass that is 25.05 parts per million less than the kilogram. Given this small, 25 ppm difference, and the fact that the mass of the IPK was indistinguishable from the mass of the Kilogramme des Archives, speaks volumes of the scientists’ skills in 1795–1799 when making their measurements of water’s properties and in manufacturing the Kilogramme des Archives.

International Prototype Kilogram (“IPK”) next to an inch-based ruler for scale

The IPK is made of a platinum-iridium alloy 

it is stored in a vault at the BIPM in Sèvres, France

Stability of the International Prototype Kilogram

By definition, the error in the measured value of the IPK’s mass is exactly zero; the IPK is the kilogram. However, any changes in the IPK’s mass over time can be deduced by comparing its mass to that of its official copies stored throughout the world, a process called “periodic verification.” For instance, the U.S. owns three kilogram replicas, two of which, K4 and K20, are from the original batch of 40 replicas of the IPK delivered in 1884. The K20 replica was designated as the primary national standard of mass for the U.S. Both of these, as well as those from other nations, are periodically shipped back to the BIPM for verification.

Note that the masses of the replicas are not precisely equal to that of the IPK; their masses are calibrated and documented as offset values. For instance, K20, the U.S.’s primary standard, originally had an official mass of 1 kg – 39 µg in 1889; that is to say, K20 was 39 µg less than the IPK. A verification performed in 1948 showed a mass of 1 kg – 19 µg. The latest verification performed in 1999 shows a mass identical to its original 1889 value. The mass of K4, the U.S.’s check standard, as of 1999 was officially calibrated as 1 kg – 116 µg. However, it was 41 µg more massive (in comparison to the IPK) in 1889.

Since the IPK and its replicas are stored in air (albeit under two or more nested bell jars), they adsorb atmospheric contamination onto their surfaces and gain mass. Accordingly, they are cleaned in preparation for periodic verifications—a process the BIPM developed between 1939 and 1946 known as “the BIPM cleaning method” that includes steam cleaning, lightly rubbing with chemical-soaked chamois, and allowing the prototypes to settle for 7–10 days. Cleaning the prototypes removes between 5 and 60 µg of contamination depending largely on the time elapsed since the last cleaning. Further, a second cleaning can remove up to 10 µg more. After cleaning—even when they are stored in their bell jars—the IPK and its replicas immediately begin gaining mass again. The BIPM even developed a model of this gain and concluded that it averaged 1.11 µg per month for the first 3 months after cleaning and then decreased to an average of about 1 µg per year thereafter. Since check standards like K4 are not cleaned before every routine calibration—a precaution to minimize the potential for wear and handling damage—the BIPM’s model has been used as an “after cleaning” correction factor.

Because the first forty official copies are made of precisely the same alloy as the IPK and are stored under similar conditions, periodic verifications using a large number of replicas—especially, the national primary standards, which are rarely used—can convincingly demonstrate the stability of the IPK. What has become clear after the third periodic verification performed between 1988 and 1992, is that for some unknown reason the mass of the IPK lost perhaps 50 µg over the last century, and possibly significantly more, in comparison to its official copies. Further, the IPK exhibits an instability of about 30 µg over a period of about a month in its after-cleaned mass. The precise reason for this instability is not fully understood but is thought to entail surface effects: microscopic differences in their polished surfaces, unintentional differences in the cleaning process, and/or differences in the precise nature of the contamination. What is known is the past assumption that the cleaning process reliably restores the prototypes to their original value is false and the BIPM’s after-cleaning correction factor is useful only for long-term trends. Scientists are seeing far greater variability in the prototypes than previously believed. Further, there is no technical means available to know whether or not the entire worldwide ensemble of prototypes suffer from even greater long-term trends upwards or downwards because their mass “relative to an invariant of nature is unknown at a level below 1000 µg over a period of 100 or even 50 years.”

The apparent loss of mass and the instability in the IPK has prompted research into improved methods to obtain a smooth surface finish using diamond-turning on newly manufactured replicas and has intensified the search for a new definition of the kilogram. 

Importance of the kilogram

As the SI system of measurement is currently defined and structured, the stability of the kilogram is crucial since it effectively underpins the entire system. For instance, the newton—the SI unit of force—is defined as the force necessary to accelerate the kilogram by one meter per second². Accordingly, if the mass of the IPK were to change slightly, so too must the newton by a proportional degree so the acceleration remains at precisely one meter/second². In turn, the pascal—the unit of pressure—is defined in terms of the newton. This chain of dependency follows to all the electrical units. For instance, the joule, which is the electrical and mechanical unit of energy, is defined as the energy expended when a force of one newton acts through one meter. The ampere too is defined relative to the kilogram. With two of the primary units of electricity thus defined in terms of the kilogram, so too follow all the rest, including the watt, volt, ohm, coulomb, farad, and weber.

Clearly, having the magnitude of many of the units comprising the SI system of measurement ultimately defined by the mass of a single, golf ball-size piece of metal that was manufactured 128 years ago is a tenuous state of affairs. The quality of the IPK must be fanatically protected in order to preserve the integrity of the SI system. Fortunately, definitions of the SI units are quite different from their practical realizations. For instance, the meter is defined as the distance light travels in a vacuum during a time interval of 1/299,792,458 of a second. However, the meter’s practical realization typically takes the form of a helium-neon laser, and the meter’s length is delineated—not defined—as 1,579,800.298 728 wavelengths of light from this laser. Note that the redefinition of the meter in terms of a duration of one second reduced the uncertainty in the wavelength of the laser light. Now suppose that the official measurement of the second was found to have drifted a few parts per billion (it’s actually exquisitely stable). There would be no automatic effect on many of the SI units of measurement because, as with the meter, the duration of the second is often abstracted through other physical principles underlying their practical realizations. Scientists performing meter calibrations would simply continue to measure out the same number of laser wavelengths until an agreement was reached to do otherwise. The same is true with regard to the real-world dependency on the kilogram: if the mass of the IPK was found to have changed slightly, there would be no automatic effect upon the other units of measure because their practical realizations provide an insulating layer of abstraction. Any discrepancy would eventually have to be reconciled though because the virtue of the SI system is its precise mathematical and logical harmony amongst its units. If the IPK’s value was found to have changed, one quick fix would be to simply redefine the kilogram as being equal to the IPK plus an offset value, similarly to what is currently done with its replicas; e.g., “the kilogram is equal to the mass of the IPK + 42 µg.”

The long-term solution is to liberate the SI system’s dependency on the IPK by developing a practical realization of the kilogram that can be reproduced in different laboratories by following a written specification. The units of measure in such a practical realization would have their magnitudes precisely defined and expressed in terms of fundamental physical constants. While major portions of the SI system would still be based upon the kilogram, the kilogram would in turn be based upon invariant, universal constants of nature. While this is a worthwhile objective and much work towards that end is ongoing, no alternative to date has achieved the uncertainty of a few parts in 10⁸ required to compete with the IPK. The most promising contender, the NIST’s implementation of the watt balance, as of late 2007 was approaching the level where scientists could resolve a difference of about 25 µg. 

Mass vs. weight

The distinction between the two

As stated above in The nature of mass, the kilogram is a unit of mass, which is an inertial property. Inertia is the property one senses when they rest a bowling ball on a level, smooth surface and forcefully push on it horizontally to accelerate it. This is quite distinct from “weight,” which is the downwards gravitational force of the bowling ball that one must counter when holding it off the floor. Unless relativistic effects apply, mass is an unchanging, universal property of matter that is unaffected by gravity. Weight on the other hand, is a property of matter that is entirely dependent upon the strength of gravity. For instance, an astronaut’s weight on the Moon is one-sixth of that on the Earth whereas his mass has changed little during the trip. Consequently, wherever the physics of recoil kinetics (mass, velocity, inertia, inelastic and elastic collisions) dominate and the influence of gravity is a negligible factor, the behavior of objects remain consistent even where gravity is relatively weak. For instance, billiard balls on a billiards table would scatter and recoil with the same speeds and energies after a break shot on the Moon as on Earth; they would however, drop into the pockets much slower.

In scientific and engineering contexts, the terms “mass” and “weight” are rigidly defined as separate measures in order to enforce clarity and precision. In everyday use, given that all masses on Earth have weight and this relationship is usually highly proportional, “weight” often serves to describe both properties, its meaning being dependent upon context. For example, the “net weight” of retail products in the U.S., which may be given in both pounds and kilograms, refers to mass. Conversely, the “load index” rating on automobile tires (Tire code), which specifies the maximum structural load for a tire in kilograms, actually refers to weight; that is, the force due to gravity.

The unit of weight: kilogram-force

When an object's weight (its gravitational force) is expressed in kilograms, the unit of measure is not a true kilogram; it is the kilogram-force (kgf or kg-f), also known as the kilopond (kp), which is a non-SI unit of force that is typically used as a unit of weight. All objects on Earth are subject to a gravitational acceleration of approximately 9.8 m/s². The CGPM (also known as the “General Conference on Weights and Measures”) fixed the value of standard gravity at precisely 9.80665 m/s² so that disciplines such as metrology would have a standard value for converting units of defined mass into defined forces and pressures. In fact, the kilogram-force is defined as precisely 9.80665 newtons. As a practical matter, gravitational acceleration (symbol: g) varies slightly with latitude, elevation and subsurface density; these variations are typically only a few tenths of a percent. See also Gravimetry.

Since masses are rarely measured to an uncertainty of better than one percent, it is technically just as valid to state that a one-kilogram object on Earth has a weight of one kilogram-force as it is to state that it has a mass of one kilogram. Accordingly, it may correctly be assumed that when someone speaks or writes of a “weight” in kilograms, they are referring to the gravitational load of the kilogram and the proper “kilogram-force” is implied.

Converting mass to force

Unlike laypeople, professionals in virtually all SI-unit-using engineering and scientific disciplines involving accelerations and kinetic energies rigorously maintain the distinctions between mass, force, and weight, as well as their respective units of measure. Engineers in disciplines involving weight loading, such as structural engineering, first convert loads due to objects like concrete and automobiles—which are always tallied in kilograms—to newtons before continuing with their calculations. Primarily, this is because material properties like elastic modulus are quite properly measured and published in terms of newtons and pascals (which is a unit of pressure derived from the newton). Kilograms are converted to newtons by multiplying by 9.8, 9.81, or 9.80665. Note that the highest-precision figure would likely result in false precision if the final product was expressed to six significant digits since loads in kilograms are rarely known with such accuracy and local gravity is rarely identical to standard gravity.

Buoyancy and “conventional mass”

The masses of objects are relatively invariant whereas their weights vary slightly with changes in barometric pressure, such as with changes in weather and altitude. This is because objects have volume and therefore have a buoyant effect in air. Buoyancy—a force that counters gravity’s—reduces the weight of all objects. Further, objects with precisely the same mass but with different densities displace different volumes and therefore have different buoyancies and weights. Normally, the effect of air buoyancy is too small to be of any consequence in normal day-to-day activities. In metrology however, where mass standards are calibrated with extreme accuracy, buoyancy is a significant effect so air density is precisely accounted for during calibration.

Given the extremely high cost of platinum-iridium mass standards, high-quality “working” standards are made of special stainless steel alloys, which occupy greater volume than those made of platinum-iridium. For convenience, a standard value of buoyancy relative to stainless steel was developed for metrology work and this results in the term “conventional mass.” Conventional mass is defined as follows: “For a mass at 20 °C, ‘conventional mass’ is the mass of a reference standard of density 8000 kg/m³ which it balances in air with a density of 1.2 kg/m³.” The effect is a small one, 150 ppm for stainless steel mass standards, but the appropriate corrections are made during the calibration of all precision mass standards so they have the true mass indicated on them. In routine laboratory use however, the reading on a precision scale when a stainless steel standard is placed upon it is actually its conventional mass; that is, its true mass minus buoyancy. Also, any object compared to a stainless steel mass standard has its conventional mass measured; that is, its true mass minus some (usually unknown) degree of buoyancy.

The effect of buoyancy invalidates the standard answer to the childhood riddle of “Which weighs more, a ton of lead or a ton of feathers (or aluminum)?” The standard answer is that they both weigh the same, but the correct answer is “Lead weighs more than aluminum, by 327 grams-force or 3.21 newtons (a difference of 0.0327%).” This is because the density of lead is greater and displaces less air.

Types of scales and what they measure

It’s notable at a purely technical level, that whenever someone stands on a balance-beam scale at a doctor’s office, they are really and truly having their mass measured. Excluding buoyancy, which affects all types of scales in fluids, balance-beam scales compare the mass on the platform with those of the sliding counterweights on the beams; gravity serves only as the force-generating mechanism that allows the needle to diverge from the “balanced” (null) point. On scales such as these, gravity can vary in strength without affecting the reading. Conversely, whenever someone steps onto a spring-based or digital load cell-based scale, they are technically having their weight measured notwithstanding that the displayed units of measure are in kilograms. On force-measuring instruments such as these, variations in gravity will affect the reading.

THE POUND

The pound or pound-mass (abbreviations: lb, lbm, or sometimes in the United States,) is a unit of mass (sometimes called 'weight' in everyday parlance) in a number of different systems, including English units, Imperial units, and United States customary units. Its size can vary from system to system. The most commonly used pound today is the international avoirdupois pound.

The distinction between mass and weight (or force) is discussed in the article on weight. In some circumstances, pound is used as the name of a unit of force. That usage is discussed in the article on pound-force, a unit of force equal to the weight of a 1-pound (approximately 0.0311 slug) mass in the standard gravitational field at Earth's surface, g. The actual gravitational field at Earth's surface varies, but the standard gravitational acceleration is usually taken to be 32.174 ft/s².

International pound

The international avoirdupois pound is equal to exactly 453.59237 grams. The definition of the international pound was agreed by the United States and countries of the Commonwealth of Nations in 1958.

In the United Kingdom, the use of the international pound was implemented in the Weights and Measures Act 1963.

"The yard or the metre shall be the unit of measurement of length and the pound or the kilogram shall be the unit of measurement of mass by reference to which any measurement involving a measurement of length or mass shall be made in the United Kingdom; and- (a) the yard shall be 0·9144 metre exactly;(b) the pound shall be 0·453 592 37 kilogram exactly.”

Weights and Measures Act, 1963, Section 1(1)

An avoirdupois pound is equal to 16 avoirdupois ounces and to exactly 7,000 grains. The conversion factor between the kilogram and the international pound was therefore chosen to be divisible by 7, and an (international) grain is thus equal to exactly 64.79891 milligrams.

Historical origin

The pound as a name for a unit of weight has a long history.

The word “pound” comes from the Latin word pendere, meaning “to weigh”. The Latin word libra means “scales, balances" and it also describes a Roman unit of mass similar to a pound. This is the origin of the abbreviation “lb” or “℔” for the pound. The plural form of “lb” is the same. Though it is frequently written with an “s” at the end (lbs), this is generally considered grammatically incorrect.

In the United Kingdom there is a historical link between the pound as a unit of mass and the pound as a unit of currency (the pound sterling), because the unit of currency was defined in the past in terms of a specific quantity of silver.

The avoirdupois pound was invented by London merchants in 1303.

The troy pound takes its name from the French market town of Troyes in France where English merchants traded at least as early as the time of Charlemagne (early ninth century). The system of Troy weights was used in England by apothecaries and jewelers.

Prior to a change during the reign of Henry VIII of England , the avoirdupois pound was based on independent standards which had been measured as about 7,002 troy grains.

During the reign of Henry VIII of England, the avoirdupois pound was redefined as 7,000 troy grains. Since then, the grain has often been considered as a part of the avoirdupois system.

In the United Kingdom, the avoirdupois pound was defined as a unit of mass by the Weights and Measures Act of 1878, but having a very slightly different value (in relation to the kilogram) than it does now, of approximately 0.453592338 kg. (This was a measured quantity, with the independently maintained artifact still serving as the official standard for this pound.) This old value is sometimes called the imperial pound, and this definition and terminology are obsolete unless referring to the slightly-different 1878 definition.

In the United States, the (avoirdupois) pound as a unit of mass has been officially defined in terms of the kilogram since 1893. In 1893, the relationship was specified to be 2.20462 pounds per kilogram. In 1894, the relationship was specified to be 2.20462234 pounds per kilogram. This change followed a determination of the British pound. The current international pound differs from the United States 1894 pound by approximately one part in 10 million.

Manual Conversion:
To convert from Pounds to Kilograms, simply multiply the Pounds by 0.45
To convert from Kilograms to Pounds, simply multiply the Kilograms by 2.2

POUNDS to KILOGRAMS

Formula x 0.4536

KILOGRAMS to POUNDS

Formula x 2.205

LINKS and REFERENCE

• Mile and Kilometre

• The U.K.’s National Physical Laboratory (NPL): FAQs on the kilogram and alternatives to the IPK

• NPL: Avogadro Project

• NIST: Hysteresis and Related Error Mechanisms in the NIST Watt Balance Experiment (888 kB PDF, here)

• BIPM home page: www.bipm.org

• NZZ Folio: What a kilogram really weighs.

• New Scientist: World's most sensitive scales weigh a zeptogram

• BIPM: The IPK in three nested bell jars

• NIST: K20, the US National Prototype Kilogram

• BIPM: Steam cleaning a 1 kg prototype before a mass comparison

• BIPM: The IPK and its six sister prototypes their vault

• NIST: The Rueprecht Balance, Austrian-made, precision balance used by the NIST from 1945 until 1960.

• BIPM: The FB-2 flexure-strip balance, the BIPM’s modern precision balance featuring a standard deviation of one ten-billionth of a kilogram (0.1 µg).

• BIPM: Mettler HK1000 balance, featuring 1 µg resolution. Also used by NIST and Sandia National Laboratories’ Primary Standards Laboratory

• Conversion Calculator for Units of SPEED

• http://www.metric-conversions.org/

• Yahoo Conversion Calculator.

• Online Pound Conversion

• U.S. National Institute of Standards and Technology Special Publication 811

• National Institute of Standards and Technology Handbook 130

Healthier alternative tastes for adventure capitalists

Solar Red | Solar Crush + | Solar Cola | Solar Citrus + | Solar +

This website is Copyright © 1999 & 2007  NJK.   The bird logo and name Solar Navigator are trademarks. All rights reserved.  All other trademarks are hereby acknowledged.       Max Energy Limited is an educational charity.

AUTOMOTIVE  |  BLUEBIRD  |  ELECTRIC CARS  |  ELECTRIC CYCLES |  SOLAR CARS